Calculating an Order-168
 Reflection Group

by Steven H. Cullinane Sept. 14, 2009

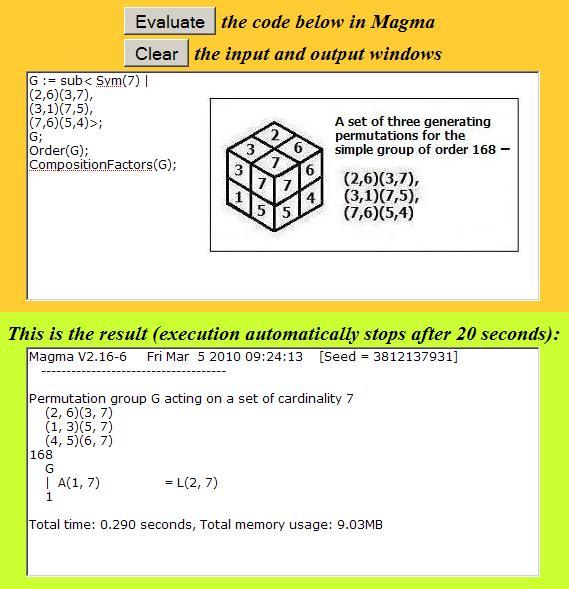
The image below is an illustration for
"A Reflection Group of Order 168"
and "The Eightfold Cube."

The calculation indicates that Klein's
simple group of order 168 is generated
by permuting parallel 1x1x2 "bricks" in
each of three halves of a 2x2x2 cube.
Each such permutation is a reflection:
a linear transformation of a vector space
that fixes a hyperplane pointwise.

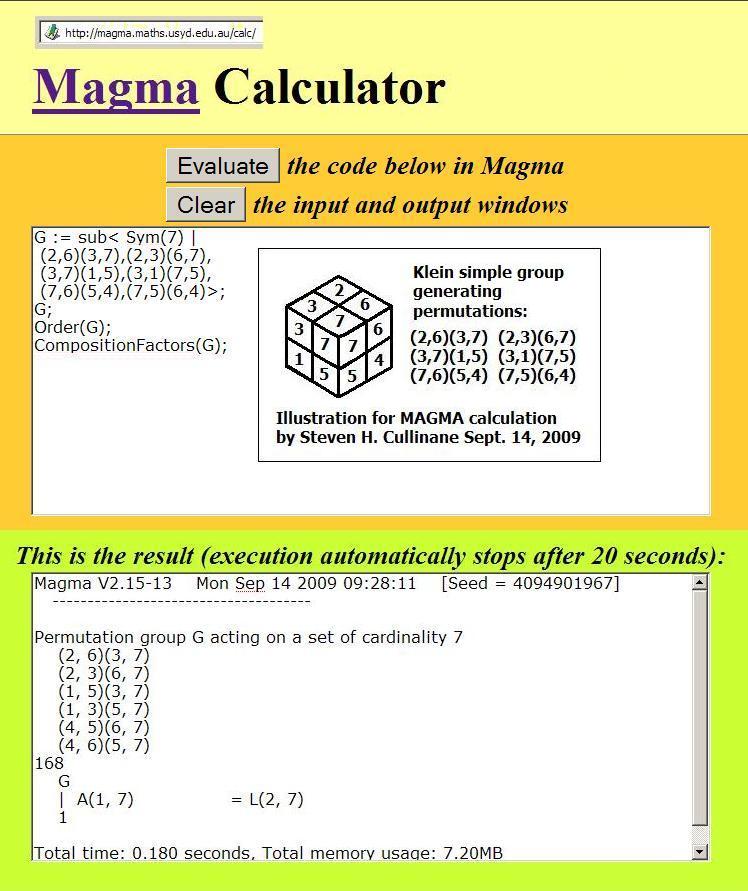
Here "A(1, 7)" is a MAGMA designation
for the group L₂(7) (Atlas notation),
which is isomorphic to the group L₃(2).
The group action illustrated by the permutations
in the calculation is of course that of L₃(2).

Note of March 4, 2010:

The group L₃(2) can be generated by using
only three of the six permutations above--