| This site gives a new arrangement of the 64 hexagrams of the I Ching. The new arrangement lets us describe the natural group of transformations of these hexagrams in a simple way. This arrangement was discovered during an investigation of the six-dimensional affine space over the two-element field by S. H. Cullinane on January 6, 1989. |
|
|
Geometry of the I Ching |
| Introduction - Hesse on the I Ching and on the chessboard: |
| "Once
Knecht confessed to his teacher that he wished to learn enough to be
able to incorporate the system of the I Ching into the Glass Bead
Game." - Magister Ludi (The Glass Bead Game), by Hermann Hesse (1943), tr. by Richard and Clara Winston, Bantam paperback, 1970, p. 117 "Here and there in the
ancient literatures we encounter legends of wise and mysterious games
that were conceived and played by scholars, monks, or the courtiers of
cultured princes. These might take the form of chess games in which the
pieces and squares had secret meanings in addition to their usual
functions." |
| Hermann
Hesse and Glass Bead Game Design Das Glasperlenspiel |
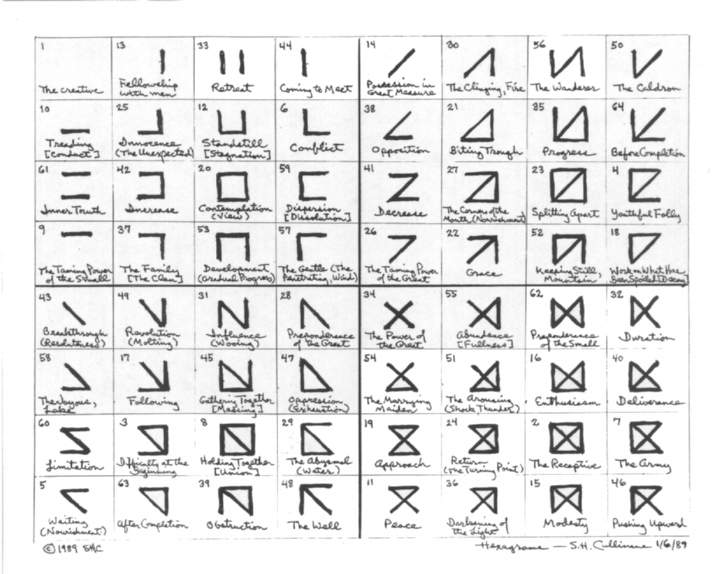
| The Cullinane sequence of the 64 hexagrams: |
| 01 13 33
44 14 30 56 50 10 25 12 06 38 21 35 64 61 42 20 59 41 27 23 04 09 37 53 57 26 22 52 18 43 49 31 28 34 55 62 32 58 17 45 47 54 51 16 40 60 03 08 29 19 24 02 07 05 63 39 48 11 36 15 46 |
| The above system of coordinates gives a 3-dimensional Karnaugh map if we interpret the four 4x4 quadrants of the 8x8 array, counted clockwise from the upper left, as the four layers, counted top to bottom, of a 4x4x4 cube. Then (with identification of opposite sides of the overall cube) each subcube is bordered by six other subcubes, each of which differs from the given subcube in exactly one coordinate. |
| Explanation of Karnaugh maps Karnaugh maps/Carroll diagrams, by Greg Gamble A (Lewis) Carroll diagram - predecessor of the 1953 Karnaugh maps |
| The I Ching as affine coordinates in a 4x4x4 cube: |
| The above labeling of the 64 subcubes of a 4x4x4 cube enables us to describe the natural transformations of the I Ching hexagrams in a geometrically simple way. See the following research note on affine groups and references on affine geometry. |
| Affine Groups on Small
Binary Spaces, by S. H. Cullinane Affine geometries compared with other geometries (from Cut-the-Knot) Ordering information for Geometry and Symmetry, by Paul B. Yale |
| The von Franz style of drawing hexagrams: |
| An
alternative way of drawing the I Ching hexagrams is suggested by
Marie-Louise von Franz. Draw a figure of six lines made up of the four
sides and the two diagonals of a square. "They are the same six lines that exist in the I Ching.... Now observe the square more closely: four of the lines are of equal length, the other two are longer.... For this reason symmetry cannot be statically produced and a dance results." - Marie-Louise von Franz, Number and Time (1970), Northwestern U. Press paperback, 1979, p. 108 One may identify the
above six lines with the yin (broken) lines of I Ching hexagrams as
follows: |
| Ordering information for books by Marie-Louise von Franz |
| References on the I Ching: |
| Related sites by S. H. Cullinane: |
Note of January 6, 1989 showing
the 64 hexagrams in the von Franz style,
in the arrangement discovered by S. H. Cullinane:
|
|
| Update of June 30, 2006: The sixteen figures in the lower right quadrant of the von-Franz-style 8x8 array above also appear in a discussion of the work of Charles Sanders Peirce, a logician of the late nineteenth and early twentieth century. See "New Light on Peirce's Iconic Notation for the Sixteen Binary Connectives," by Glenn Clark, in Studies in the Logic of Charles Sanders Peirce, Indiana University Press, 1997. Peter Loly of the University of Manitoba has also investigated cubic and square arrangements of the 64 hexagrams that have the Karnaugh property (adjacent figures differing in only one place). See his paper "A Logical Way of Ordering the Hexagrams of the Yijing and the Trigrams of the Bagua" (pdf), published in The Oracle-- The Journal of Yijing Studies, vol. 2, no. 12, January 2002, pp. 2-13. The new illustration below may serve to clarify some of my earlier remarks above and to show how Loly's "unfolding" process may be applied to my 1989 arrangement to yield a flat "chessboard" of hexagrams that, like the cube, has the Karnaugh property.  |
| Page
last updated June 30, 2006; created August 8, 2000. |